Discrete-time Fourier transform (DTFT)
In the last two posts in my Fourier transform series I discussed the continuous-time Fourier transform. Today I want to start getting "discrete" by introducing the discrete-time Fourier transform (DTFT).
The DTFT is defined by this pair of transform equations:

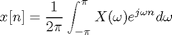
Here x[n] is a discrete sequence defined for all n:
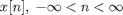
I am following the notational convention (see Oppenheim and Schafer, Discrete-Time Signal Processing) of using brackets to distinguish between a discrete sequence and a continuous-time function. n is unitless. The frequency-domain variable,  , is continuous with units of radians.
, is continuous with units of radians.
Note that  is periodic with period
is periodic with period  .
.
Here are a few common transform pairs:
Unit Impulse
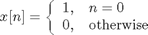

DTFT of Unit Impulse


Rectangular Pulse


DTFT of Rectangular Pulse


Note that the DTFT of a rectangular pulse is similar to but not exactly a sinc function. It resembles the sinc function between
 and
and  , but recall that
, but recall that  is periodic, unlike the sinc function.
is periodic, unlike the sinc function.
Cosine


DTFT of Cosine
The DTFT of a discrete cosine function is a periodic train of impulses:


I updated the above plot on 6-Jan-2010 to show the location of the impulses. -SE
Because of the periodicity of  it is very common when plotting the DTFT to plot it over the range of just one period:
it is very common when plotting the DTFT to plot it over the range of just one period: 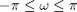 . For example, the DTFT of the rectangular pulse will most often be shown like this:
. For example, the DTFT of the rectangular pulse will most often be shown like this:

Next time I'll discuss the relationship between the continuous-time and the discrete-time Fourier transforms. Until then, Happy New Year everyone!
- Category:
- Fourier transforms
 Cleve’s Corner: Cleve Moler on Mathematics and Computing
Cleve’s Corner: Cleve Moler on Mathematics and Computing The MATLAB Blog
The MATLAB Blog Guy on Simulink
Guy on Simulink MATLAB Community
MATLAB Community Artificial Intelligence
Artificial Intelligence Developer Zone
Developer Zone Stuart’s MATLAB Videos
Stuart’s MATLAB Videos Behind the Headlines
Behind the Headlines File Exchange Pick of the Week
File Exchange Pick of the Week Hans on IoT
Hans on IoT Student Lounge
Student Lounge MATLAB ユーザーコミュニティー
MATLAB ユーザーコミュニティー Startups, Accelerators, & Entrepreneurs
Startups, Accelerators, & Entrepreneurs Autonomous Systems
Autonomous Systems Quantitative Finance
Quantitative Finance MATLAB Graphics and App Building
MATLAB Graphics and App Building




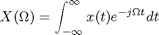


Comments
To leave a comment, please click here to sign in to your MathWorks Account or create a new one.