Fourier transforms – where to go from here
Last fall I started posting occasionally about Fourier transforms. This topic causes much confusion, which is particularly unfortunate given how many people are interested in it.
I speculate that the fundamental source of confusion is that there are really several different (but related) transforms that go by the name "Fourier transform." Most people who encounter the Fourier transform only know about one form, and the confusion starts when they encounter another form which doesn't seem to have the same properties as the one they learned.
To address that confusion, I have previously described three of the four basic forms of Fourier transform: the continuous-time Fourier transform, the discrete-time Fourier transform, and the discrete Fourier transform. And I've described the relationships between them.
Well, now it's been a few weeks since my last Fourier transform post, and I'm thinking about where to go next. I'd like to get your feedback and advice about this.
I've reviewed my own notes and brainstormed a bit, and I've also reviewed all of your posted comments. In no particular order, here are some possible follow-up topics.
- Sampling theorem and the strict inequality issue raised in a comment thread
- Positive and negative frequencies
- How to make DTFT-style plots (1-D, 2-D, and labeling in units of pi)
- How to make a frequency-domain plot labeled with physical frequency units
- How to make a frequency-domain plot labeled with other units (wavenumber, km)
- Things that surprise about the DFT (why complex? why zero-frequency at the left? why doesn't it look right for a "pure" sinusoid?)
- What's the deal with fftshift? When do I need to use it and why?
- Symmetry properties for the DFT (they're all circular!)
- Frequency-domain-based filtering
- Gibbs phenomenon and ringing
- Importance of phase information for images
- Revisit visualization techniques for Fourier transforms of images
What would you like for me to add this list? What surprises you or puzzles you about the output of the fft function? Please let me know by posting a comment.
- Category:
- Fourier transforms
 Cleve’s Corner: Cleve Moler on Mathematics and Computing
Cleve’s Corner: Cleve Moler on Mathematics and Computing The MATLAB Blog
The MATLAB Blog Guy on Simulink
Guy on Simulink MATLAB Community
MATLAB Community Artificial Intelligence
Artificial Intelligence Developer Zone
Developer Zone Stuart’s MATLAB Videos
Stuart’s MATLAB Videos Behind the Headlines
Behind the Headlines File Exchange Pick of the Week
File Exchange Pick of the Week Hans on IoT
Hans on IoT Student Lounge
Student Lounge MATLAB ユーザーコミュニティー
MATLAB ユーザーコミュニティー Startups, Accelerators, & Entrepreneurs
Startups, Accelerators, & Entrepreneurs Autonomous Systems
Autonomous Systems Quantitative Finance
Quantitative Finance MATLAB Graphics and App Building
MATLAB Graphics and App Building



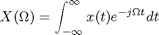
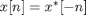


Comments
To leave a comment, please click here to sign in to your MathWorks Account or create a new one.