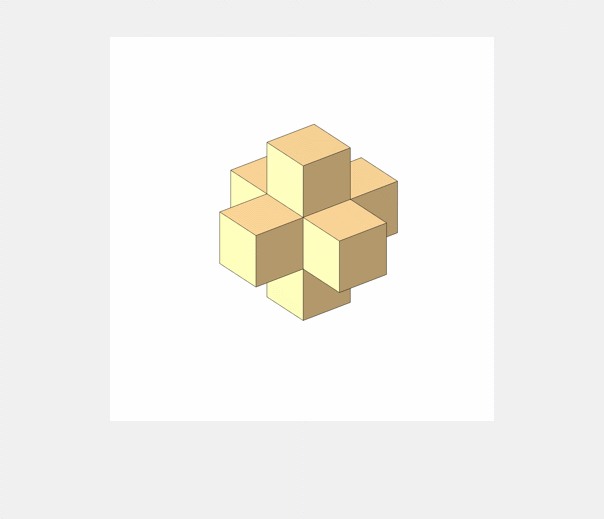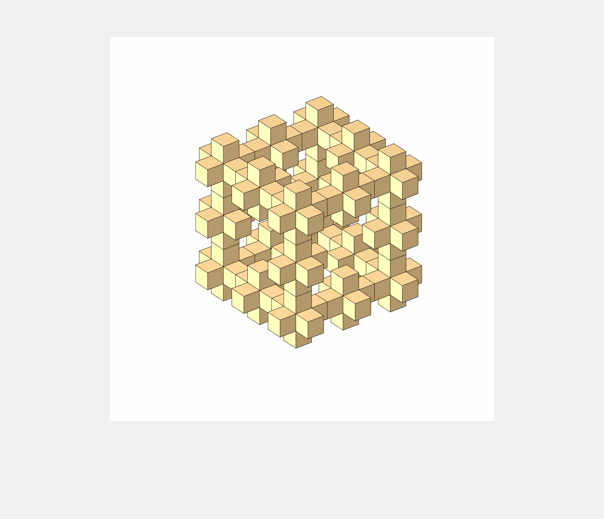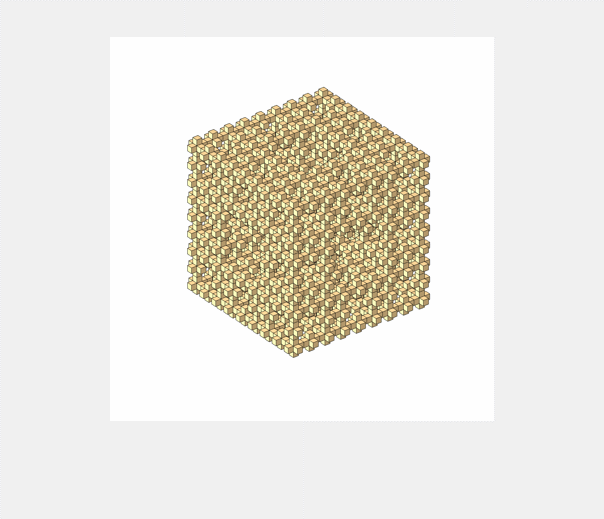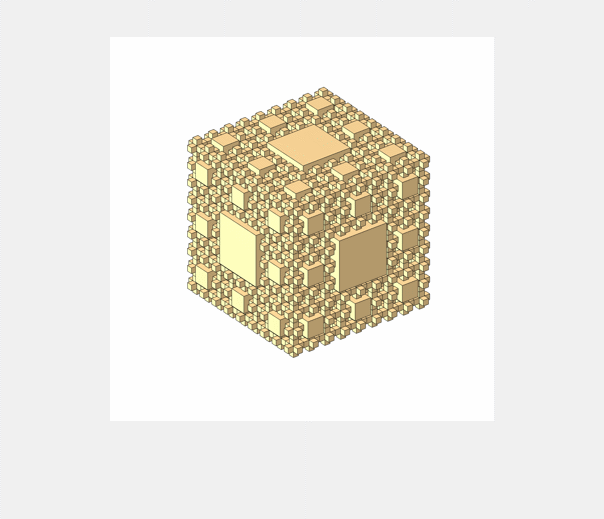
A few months ago, I had never heard of Karl Menger or the cube-like fractal that he invented. Now I have this long blog post about the sponge, and an extension that I am calling its "associate".... read more >>
The complement of an evolving Menger sponge is all of the material that has been removed so far.... read more >>
Season's Greetings 2021 __________________________________________________________Marc Lätzel (2021). XMas Tree (https://www.mathworks.com/matlabcentral/fileexchange/9337-xmas-tree),MATLAB... read more >>
The Menger sponge is a popular fractal that generalizes Cantor sets and Sierpinski triangles. Its fractal dimension is between two and three.... read more >>
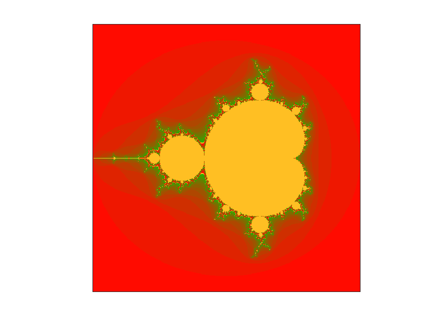
Mandelbrot Brings Season's GreetingsMandelbrot sports a new red, green and gold colormap to celebrate the holidays. old_mandelbrot holiday_mandelbrot Available in Version 4.10 of Cleve's... read more >>
Antarctic Ships, Fractal and RealDavid Wilson, from the Auckland University of Technology in New Zealand, has alerted me to this remarkable coincidence. Here is the "Burning Ship" from my post... read more >>
If you follow this blog regularly, you know that I love fractals. I recently spent a pleasant afternoon in Nashua, New Hampshire, where my daughter Teresa introduced me to Gregory Searle, a fractal artist and computer geek. Here is his logo.... read more >>
Let me tell you about a beautiful, fractal curve, the Dragon Curve. Download my new dragon program from the File Exchange and follow along.... read more >>
I don't recall where I found this seasonal fractal. And I can't explain how it works. So please submit a comment if you can shed any light on either of these questions.... read more >>
