Benford’s Law – What are the odds that the first digit is a ‘1’?
I'd like to introduce this week's guest blogger Sam Mirsky. Sam is an Application Engineer here at MathWorks who focuses on real-time testing applications using Simulink. However, in this post he will talk about a non-intuitive characteristic of large data sets, and test the idea with a data set which ships with MATLAB.
In a large set of data, it seems that the probability of individual numbers starting with 1 would be the same as any other digit. However, this is not true. There is a much higher probability that the first digit is a 1.
Since the first significant digit is not zero, the intuitive probability of a number starting with 1 (or any other digit) would be 1/9 = 11%. According to Wikipedia: "The first digit is 1 about 30% of the time, and larger digits occur as the leading digit with lower and lower frequency, to the point where 9 as a first digit occurs less than 5% of the time."
Contents
Load Data
Let us test this with a data set which ships with MATLAB: quake.mat. This is a data set with accelerometer data from an earthquake in California.
load quakeFind digit statistics
stat(1:9) = 0; for i = 1:length(v) string = sprintf('%0.5e', abs(v(i))); firstDigit = str2double(string(1)); switch firstDigit case 1 stat(1) = stat(1) +1; case 2 stat(2) = stat(2) +1; case 3 stat(3) = stat(3) +1; case 4 stat(4) = stat(4) +1; case 5 stat(5) = stat(5) +1; case 6 stat(6) = stat(6) +1; case 7 stat(7) = stat(7) +1; case 8 stat(8) = stat(8) +1; case 9 stat(9) = stat(9) +1; end end
Plot results
statPercent = stat / sum(v ~= 0); %only use non-zero numbers for stats bar(statPercent); grid on; xlabel('First digit'); ylabel('Percent');
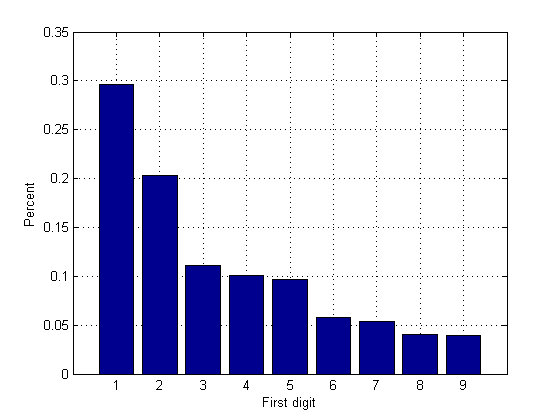
How this is used
This is one test that is done to test if a data set is real or fabricated. For example, if you collect all the numbers on a federal income tax return, it should also obey Benford's Law.
How would you use MATLAB to calculate these statistics?
As is typical with MATLAB, there are many ways to derive the same answer:
- What MATLAB commands would you use to analyze the first digit of numbers in a data set?
- Does Benford's Law apply to a data set you have (or not)? Show us your results here.
- Category:
- Fun
 Cleve’s Corner: Cleve Moler on Mathematics and Computing
Cleve’s Corner: Cleve Moler on Mathematics and Computing The MATLAB Blog
The MATLAB Blog Guy on Simulink
Guy on Simulink MATLAB Community
MATLAB Community Artificial Intelligence
Artificial Intelligence Developer Zone
Developer Zone Stuart’s MATLAB Videos
Stuart’s MATLAB Videos Behind the Headlines
Behind the Headlines File Exchange Pick of the Week
File Exchange Pick of the Week Hans on IoT
Hans on IoT Student Lounge
Student Lounge MATLAB ユーザーコミュニティー
MATLAB ユーザーコミュニティー Startups, Accelerators, & Entrepreneurs
Startups, Accelerators, & Entrepreneurs Autonomous Systems
Autonomous Systems Quantitative Finance
Quantitative Finance MATLAB Graphics and App Building
MATLAB Graphics and App Building








Comments
To leave a comment, please click here to sign in to your MathWorks Account or create a new one.